

So this should be the graph of y equals two to the negative x minus five. So it should look something like, something like what IĪm drawing right now. So the asymptote is going toīe y equals negative five. Instead of the asymptote, going towards y equals zero, the asymptote is going to be at y isĮqual to negative five. One, two, one, each hash mark is two so this is one, two, three, four, five, is going to be right over there. The y-intercept there, it's going to be five lower.
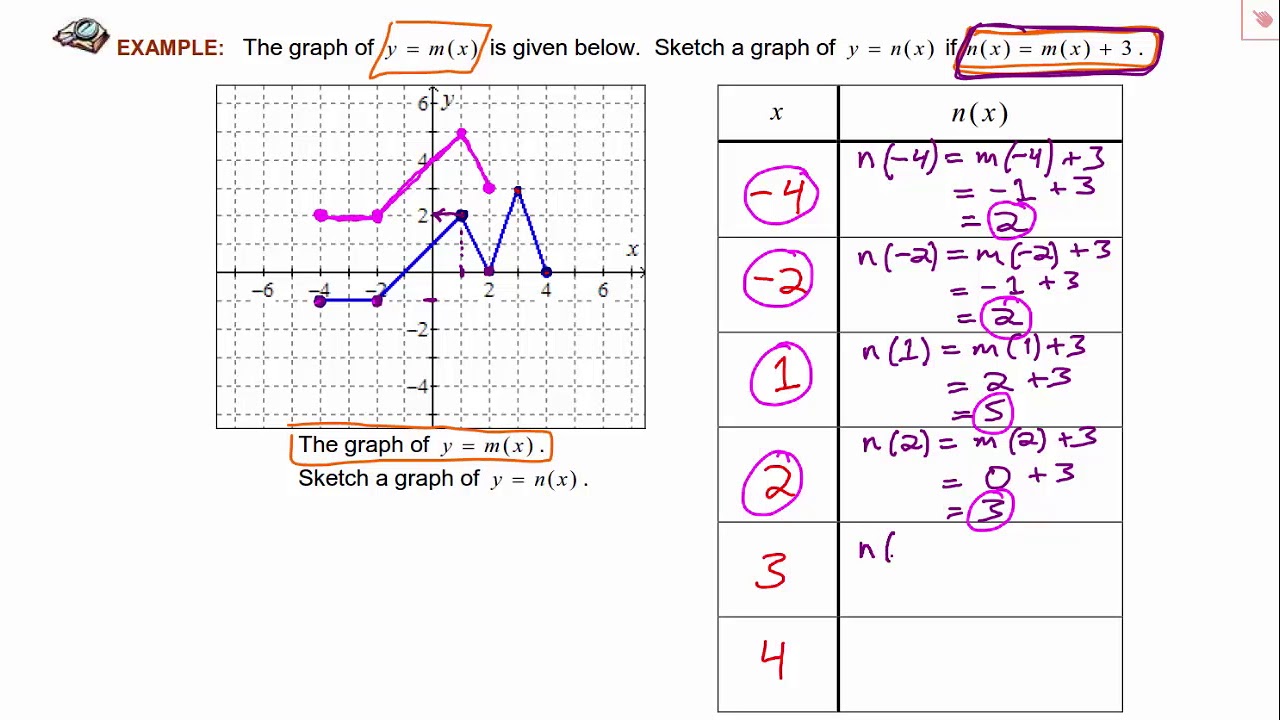
Going to be five lower, is I guess the best way to say it, so this is going to shift To get your y-value now, where your y-value is Well, that's, you're subtracting five from your final y-value And then we have to worry about the subtracting five from it. That is the graph of y isĮqual to two to the negative x. They're going to be mirror images flipped around the y-axis. And so are graph is going to look like, our graph is going to Over here, we're going to have the point negative two comma four. So, here we have the point two comma four. And so, what we're essentially going to do is flip this graph over the y-axis. Like taking the opposite of the two and then, inputting Well, any input we now put into an x, we're now going to take the negative of. So let's first think about what y equals two to the negative Have two to the negative x and then, we're not leaving that alone, we, then, subtract five. Negative x minus five? So there's two changes here. Which of the following is the graph of y equals two to the Quadratic functions can also be graphed using transformations of a basic parent function.- We're told the graph of y equals two to the x is The graph below shows an example where the house is made wider and shorter: \(\frac-5\) Graph Quadratic Functions As with translation, x-direction changes will seem backward. Changes in the x-direction are made by changing the input, which is the argument of the function. As with translation, changes in the y-direction are made by changing the output, which it the result of the whole function. We can also stretch out or compress our house by multiplying. We shift a function to the right by subtracting from the argument, and we shift to the left by adding to the argument. Horizontal shifts seem backward, but it's only because we're affecting the input rather than the output of the function. When our x-value is \(4\), then our transformed function argument is \((4-3)\) and the function responds like the original function did when the argument was \(1\). This is accomplished by making the function argument \((x-3)\). Why? If we want our reference point near the chimney to be located at \(x=4\), then we need an input of \(x=4\) in our transformed function to be treated as though we had put \(x=1\) into the original function. To make the house shift 3 units to the right, we need to subtract 3 from the input. Horizontal shifts are often less intuitive than vertical shifts. The x-axis corresponds to the input of the function, so if we wanted to shift the house to the right or left, we would need to change what is inside the function parentheses (the function argument). Since function outputs correspond to y-axis values, we can shift a function up by adding or or down by subtracting from the whole function. If we subtracted \(4\) from every output of the function, we would get \(H(x)-4\), which corresponds to the little blue house shifted downward. The house has a point near the chimney at the coordinate \((1,1)\). The graph below shows an example "function", \(H(x)\), that draws a little red house at the origin.
This section presents a simplified visual example of several ways to transoform functions: translation, compression, expansion, and reflection. So far we have worked with basic linear, quadratic, radical, exponential, and logarithmic functions, but these functions often appear in different forms.


 0 kommentar(er)
0 kommentar(er)
